Artists who breathe life into animated characters in movies and video games now have the opportunity to exert more creative control over their animations, thanks to a groundbreaking technique developed by researchers at MIT. This new method introduces mathematical functions called barycentric coordinates, which allow 2D and 3D shapes to bend, stretch, and move in space. Unlike previous techniques that provide limited options for these coordinate functions, the MIT researchers’ approach offers unprecedented flexibility. Not only does this advancement benefit the world of art and animation, but it also has promising applications in fields such as medical imaging, architecture, virtual reality, and computer vision.
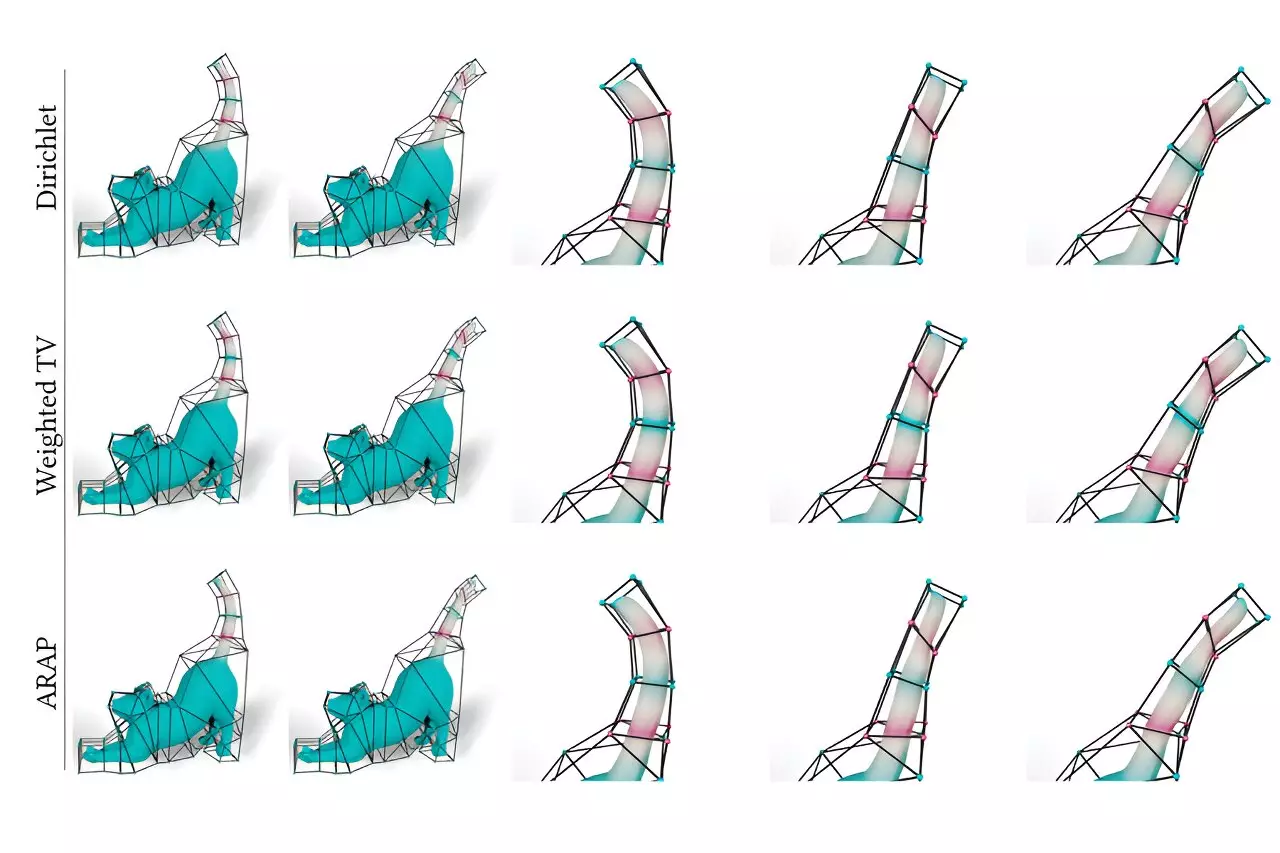
Traditionally, animators have surrounded the complex shape of a character with a simpler set of points connected by lines or triangles, known as a cage. By manipulating these points, animators can move and deform the character within the cage. The challenge, however, lies in determining how the character moves when the cage is modified—an aspect controlled by a specific barycentric coordinate function. Previous methods rely on intricate equations to ensure smooth motions without any irregularities. Yet, there are numerous interpretations of what constitutes “smoothness” in art, leading to a variety of coordinate functions. The MIT researchers aimed to develop a general approach that empowers artists to participate in designing or selecting smoothness energies for any shape, allowing them to preview and choose the deformation that best suits their vision.
The concept of barycentric coordinates dates back to the 19th century when German mathematician August Möbius first introduced it. These coordinates define how each corner of a shape influences its interior, making them relatively easy to design for triangles—the shape Möbius focused on. However, when dealing with more complex cages, the calculations become increasingly intricate. To overcome this challenge, the MIT researchers turned to neural networks, a remarkable tool inspired by the human brain. Rather than using neural networks for artificial intelligence purposes, the team employed them for their mathematical capabilities. Their network architecture consciously generates barycentric coordinate functions that satisfy all the necessary constraints, combining them to create complex yet smooth motions.
Since modern cages are typically far more complex than triangles, the team devised a method that divides the shape into adjoining virtual triangles. Each virtual triangle represents a valid barycentric coordinate function, and the neural network predicts how to combine these functions to create more intricate but seamless motions. This approach gives animators the ability to generate multiple functions, alter the coordinates, and iteratively refine their animations until they achieve the desired look. By incorporating neural networks, the researchers provide artists with newfound flexibility and creative possibilities.
The MIT researchers showcased the superiority of their technique by demonstrating how it can produce more natural-looking animations. For example, a cat’s tail that previously folded rigidly near the vertices of the cage can now curve smoothly as it moves. Looking ahead, the team plans to explore different strategies for accelerating the neural network’s performance. Additionally, they aim to integrate this method into an interactive interface that allows artists to iteratively refine their animations in real-time. Ultimately, this revolutionary technique will revolutionize the animation industry, empowering artists and giving them the tools they need to bring their creative visions to life.


Leave a Reply